Imager field of view

Figure 3: The reimaging system with the paths of light from on-axis (black lines) and off-axis objects (red and blue lines). The beams from the off-axis objects are drawn as parallel to the on-axis beam. These are called ``telecentric'' beams; real systems are not always telecentric, but the deviations from it are small for the purposes of this discussion. Note that the collimator has to be larger than a single beam to accept off-axis beams, and that all of the beams pass through a ``waist'' at the pupil.

In order to image a nonzero field of view, the collimator has to accept off-axis beams, and be physically wider than $D_{pupil}$. Figure 3 shows the path of off-axis beams through the optical system. For the simplified case in which the off-axis beams are parallel to the optical axis (``telecentric'') and we have abstracted the collimator as a simple lens, then

$$D_{coll} = D_{pupil} + D_{field}.$$

[Side note: For a complex lens, the $D_{coll}$ isn't necessarily the diameter of all of the collimator glass, but the diameter of the collimator entrance pupil. The entrance pupil of a lens by itself is not the same thing as the pupil of the whole system, indicated by the vertical dotted line. In both cases, pupil means the image of an aperture stop, but for the whole system, the aperture stop is the primary mirror.]

Note that the collimator must have total f-number

$$N_{coll} = f_{coll} / D_{coll},$$

$$N_{coll} = N_{tel} \frac{D_{pupil}}{D_{coll}},$$

$$N_{coll} = N_{tel} \frac{D_{pupil}}{D_{pupil}+D_{field}}.$$

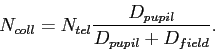
The whole collimator lens is faster than the beam delivered by the telescope, because it has to be wide enough to accept the off-axis beams. Faster lenses or mirrors that accept light from larger off-axis angles are harder to construct. Imaging over a wide field also has to contend with the curvature of the telescope focal plane. In practice, both the size of the available detector and the requirement of good image quality for off-axis points set limits on the field of view of an instrument.